80-110, the more straight lines area, the fewer waypoints.
 JeanPhilippe_Jobin
JeanPhilippe_Jobin
I agree with @BeachChE , 80-110 is a good guess.
Since there are around 30 arcs and each arc requires 3 waypoints, I would say around 90.

 BeachChE
BeachChE
 Alexandre_Pare
Alexandre_Pare
 Ryan_Weaver
Ryan_Weaver
 Olivier_Grenier
Olivier_Grenier
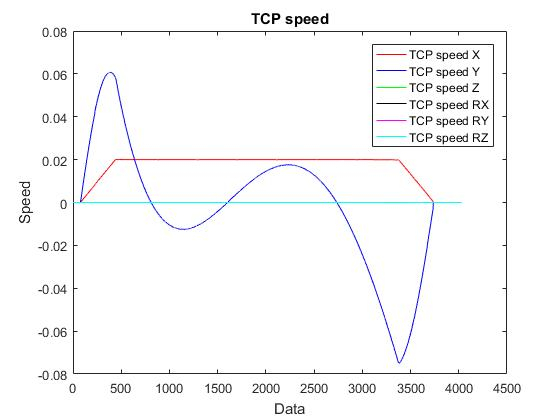

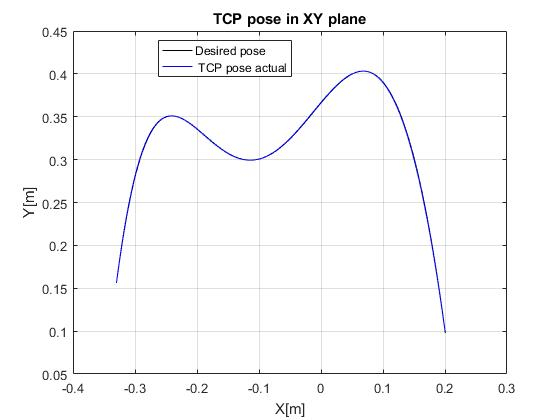
 matthewd92
matthewd92


Hello everyone,

We wanted to get your input on how you would apply a sealing material to the part below with a Universal Robots (following the red line). How many waypoints do you think it would take?
Looking forward to your answers.